The Sir Model For Spread Of Disease

Thus we have three Euler formulas of the form. The SIR model of an infectious disease The model I will introduce is the Susceptible Infected and Recovered SIR model. SIR model for COVID-19 According to this model and without any intervention to contain the spread the virus would be extinguished in about 180 days saving less than 20 of the population. More information about video. The SIR Model for Spread of Disease. The SIR Model for Spread of Disease - Relating Model Parameters to Data. S_t the number of susceptibles in day t I_t the number of infectives in day t R_t.

The Differential Equation Model.

Its basic purpose is to help us understand the way a contagious disease spreads. It examines how an infected population spreads a disease to a susceptible population which transforms into a recovered population. Initial exploration of model. Any conclusion we reach about the model can then be interpreted to tell us something about the reality. The model introduced in the video introduction contained three state variables. Its basic purpose is to help us understand the way a contagious disease spreads.

Sir Model Schematic Representation Differential Equations And Plot Download Scientific Diagram

The sir model for spread of disease. The SIR Model for Spread of Disease - The Contact Number. The SIR Model for Spread of Disease - Eulers Method for Systems. The SIR Model for Spread of Disease.

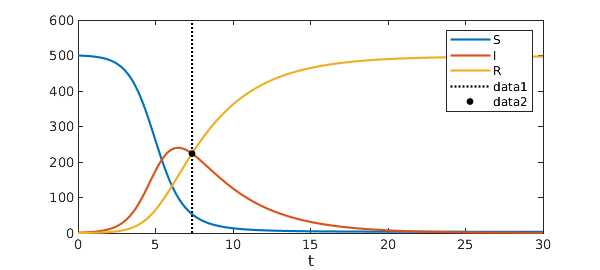
The SIR Model for Spread of Disease - Relating Model Parameters to Data. Compare your calculated value with the one you get by direct calculation from the definition c bk. The independent variable is time t measured in days.

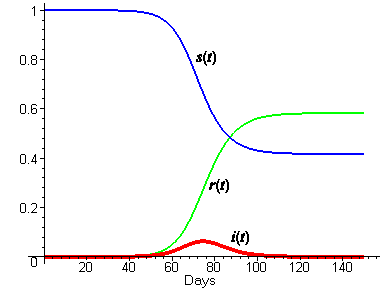
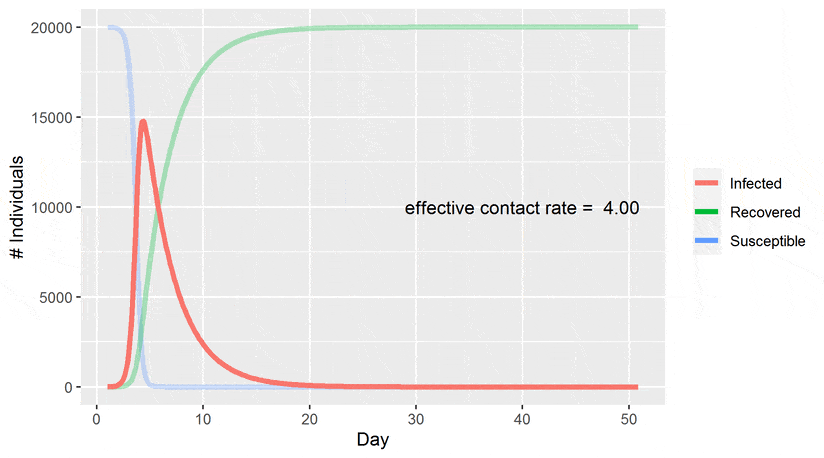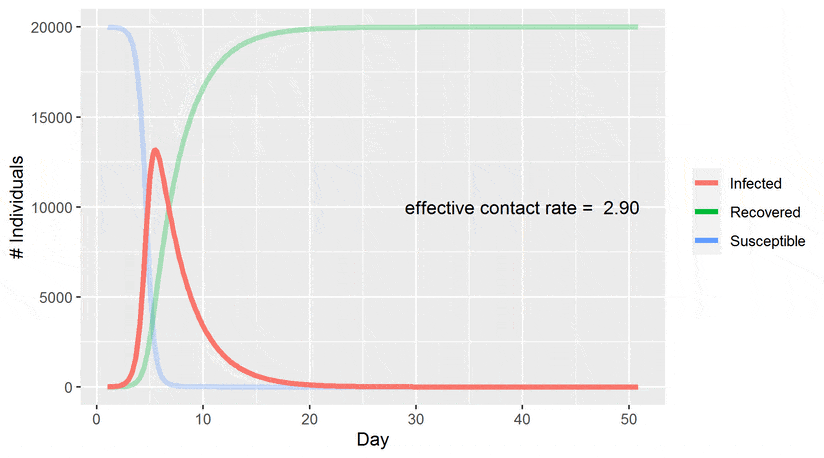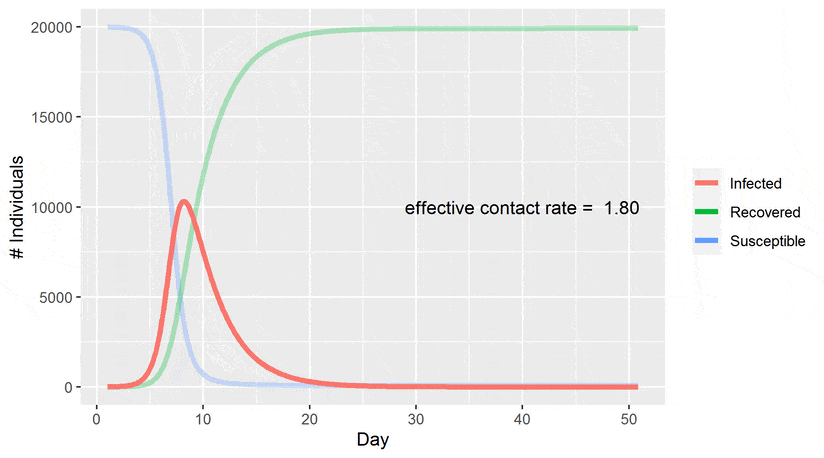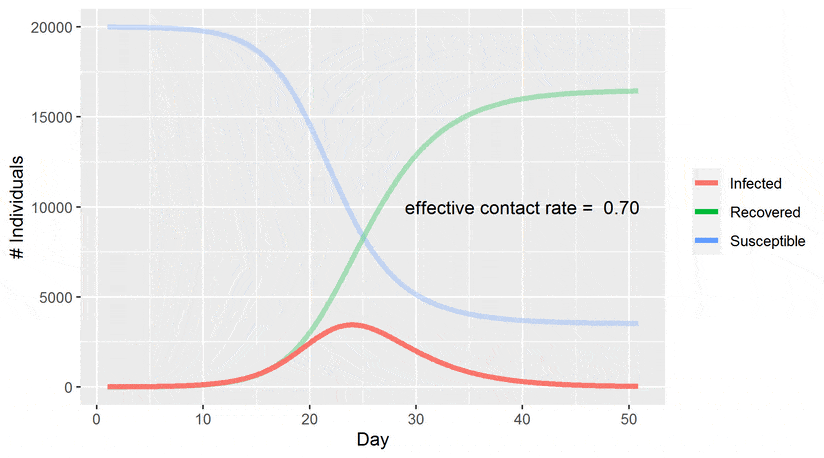
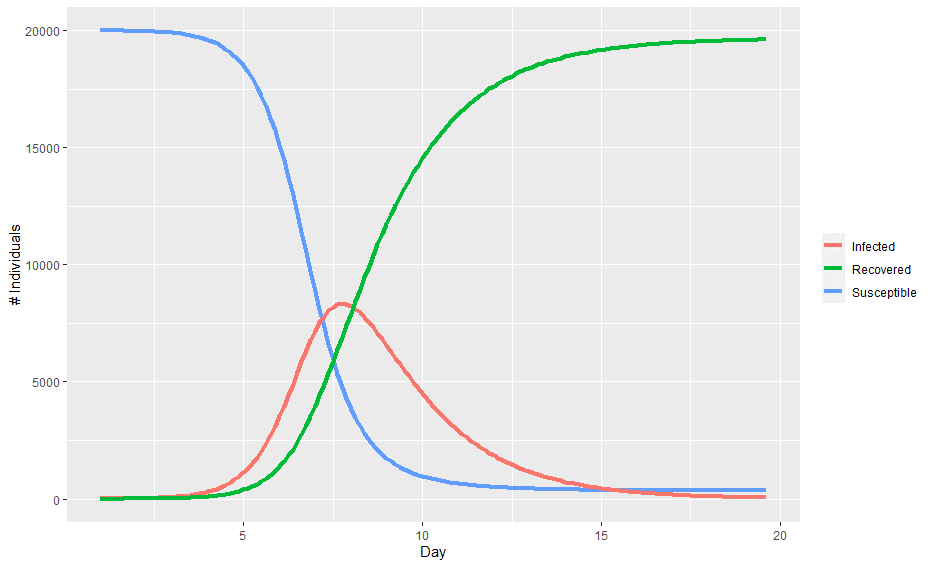
3 linhas The first set of dependent variables counts people in each of the groups each as a function of. The SIR Model for Spread of Disease - Relating Model Parameters to Data. During the winter of 1968-1969 the United States was swept by a virulent new strain of influenza named Hong Kong flu for its place of discovery.

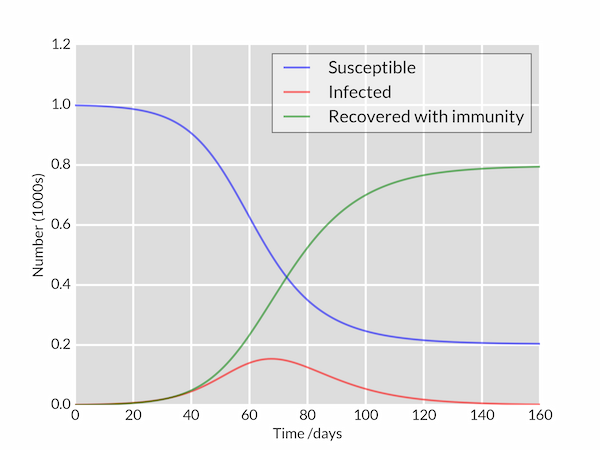
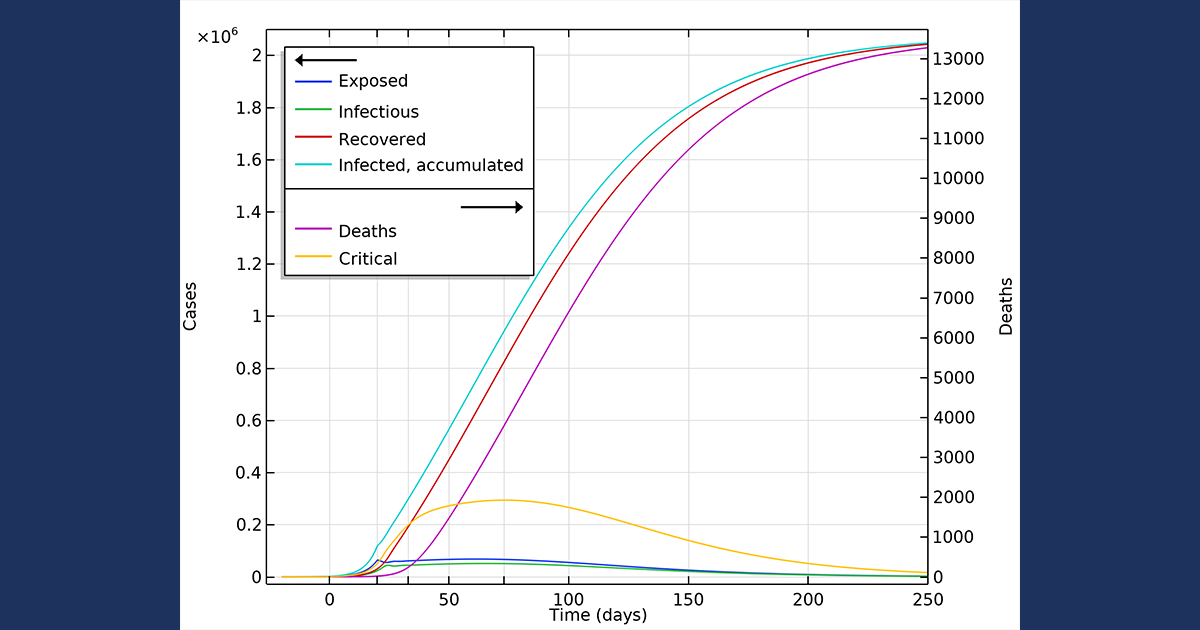
SIR model for COVID-19 According to this model and without any intervention to contain the spread the virus would be extinguished in about 180 days saving less than 20 of the population. The SIR Model for Spread of Disease. This is calculus as tool.

It examines how an infected population spreads a disease to a susceptible population which transforms into a recovered population. The use of mathematics to model the spread of infectious disease is an increasingly critical tool not just for epidemiologists and health care providers. Initial exploration of model.

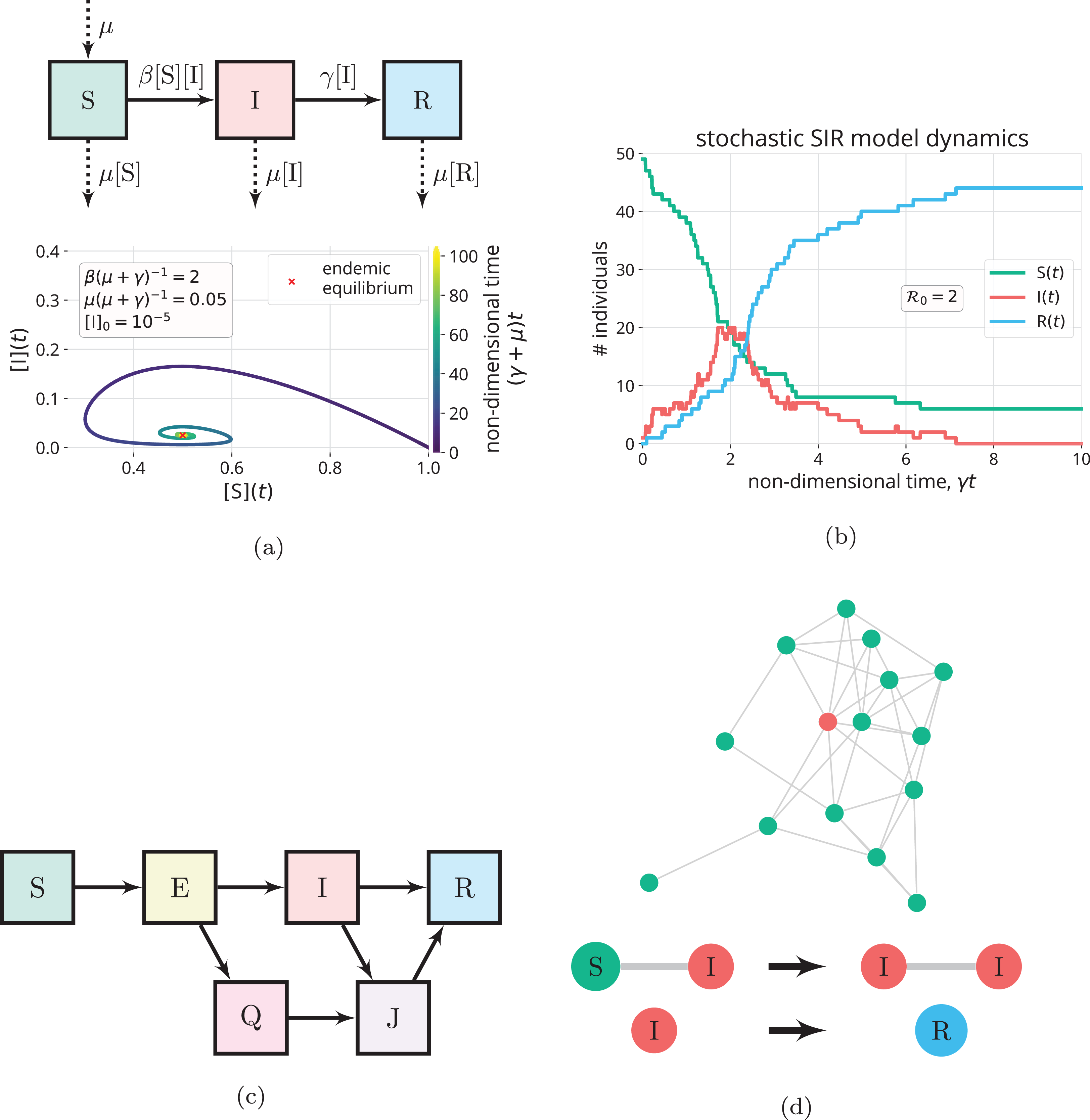
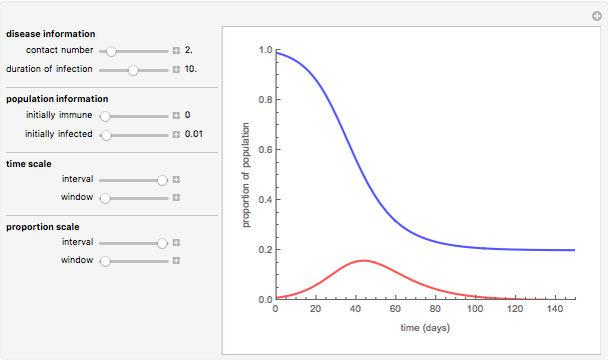
SIR model is of course a very simple model not suitable for shaping complex dynamics especially those which involve large and different populations. David Smith and Lang Moore The SIR Model for Spread of Disease - The Contact Number Convergence December 2004 JOMA. The SIR Model for Spread of Disease - Background.

The SIR Model for Spread of Disease. The SIR Model for Spread of Disease - Herd Immunity.

The Sir Model For Spread Of Disease The Differential Equation Model Mathematical Association Of America

The Sir Dynamic Model Of Infectious Disease Transmission And Its Analogy With Chemical Kinetics Peerj

Modelling Coronavirus Beyond The Sir Model Towards Data Science

The Sir Epidemic Model

Compartmental Models In Epidemiology Wikipedia

The Sir Dynamic Model Of Infectious Disease Transmission And Its Analogy With Chemical Kinetics Peerj

Modelling Infectious Disease Outbreaks Chebfun

The Sir Infectious Disease Model Preliminary Analysis Youtube

Typical Sir Model Solution Showing Progression Of Population Disease Download Scientific Diagram

Dynamic Modeling Of Covid 19 Kx

Modeling The Spread Of Infectious Disease The Sir And Mseir Compartment Models Taurus Londono S Blog

Sir Model Solving Sir Model

Compartmental Models In Epidemiology Wikipedia

Compartmental Models In Epidemiology Wikipedia

Dynamic Modeling Of Covid 19 Kx

Tracking The Early Depleting Transmission Dynamics Of Covid 19 With A Time Varying Sir Model Scientific Reports

Https Www Medrxiv Org Content 10 1101 2020 05 15 20103077v1 Full Pdf

Sir Compartment Model Covid 19 Data Science And Machine Learning Kaggle

Sir Epidemic Dynamics Wolfram Demonstrations Project

The Sir Model With Births And Deaths Application Center

Sir Model Simple English Wikipedia The Free Encyclopedia

The Challenges Of Modeling And Forecasting The Spread Of Covid 19 Pnas

Mathematical Models Of Sir Disease Spread With Combined Non Sexual And Sexual Transmission Routes Sciencedirect

1

The Sir Model For Spread Of Disease Herd Immunity Mathematical Association Of America

Modeling The Spread Of Diseases A Simulation Exercise With Sir Models By Andrea Capitanelli Towards Data Science

Video Introduction To An Infectious Disease Model Math Insight

Modelling Infectious Diseases Ib Maths Resources From British International School Phuket

Https Calculate Org Au Wp Content Uploads Sites 15 2018 10 Spread Of Disease Pdf

Modeling The Spread Of Covid 19 With Comsol Multiphysics Comsol Blog

Solved Problem 2 An Sir Model For Disease Spread In T Chegg Com

The Classic Sir Model Application Center

Sir Epidemic Models Cs 390 590 Fall Ppt Video Online Download

Covid 19 Dynamics With Sir Model The First Cry Of Atom

Sir Model Of The Impact Of Vaccine Introduction On The Course Of Download Scientific Diagram

Modelling The Covid 19 Epidemic And Implementation Of Population Wide Interventions In Italy Nature Medicine

Modelling Infectious Diseases Ib Maths Resources From British International School Phuket

The Sir Model For Spread Of Disease Augustory Corp

A Discrete Sir Infectious Disease Model Math Insight

The Mathematics Of Diseases Plus Maths Org

Https Cran R Project Org Web Packages Shinysir Vignettes Vignette Html

The Sir Model For Spread Of Disease The Differential Equation Model Mathematical Association Of America

Epidemic Modelling With Compartmental Models Using R Polymatheia

Scielo Brasil Impact Of Network Topology On The Spread Of Infectious Diseases Impact Of Network Topology On The Spread Of Infectious Diseases

Solved Problem 2 The Sir Model For Disease Spread In Th Chegg Com

How Covid 19 And Other Infectious Diseases Spread Mathematical Modeling

Epidemic Modeling 102 All Covid 19 Models Are Wrong But Some Are Useful By Bruno Goncalves Data For Science

1

Compare your calculated value with the one you get by direct calculation from the definition c bk.

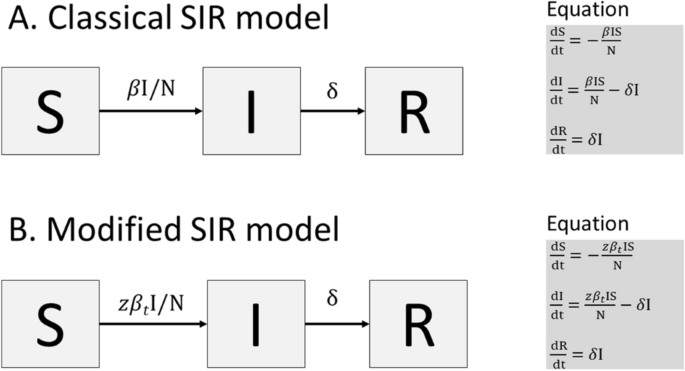
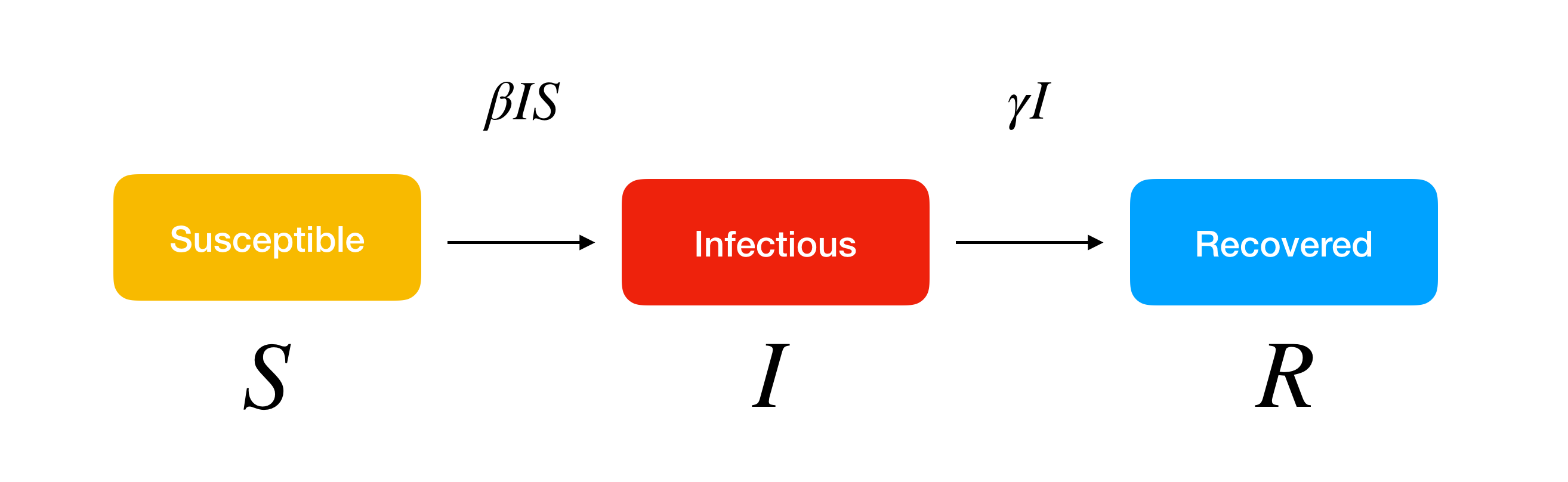
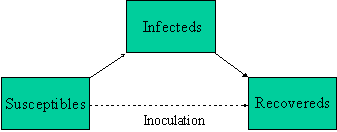
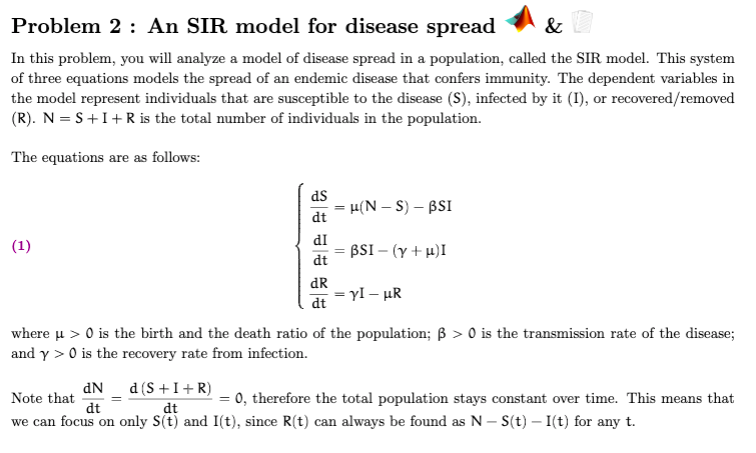
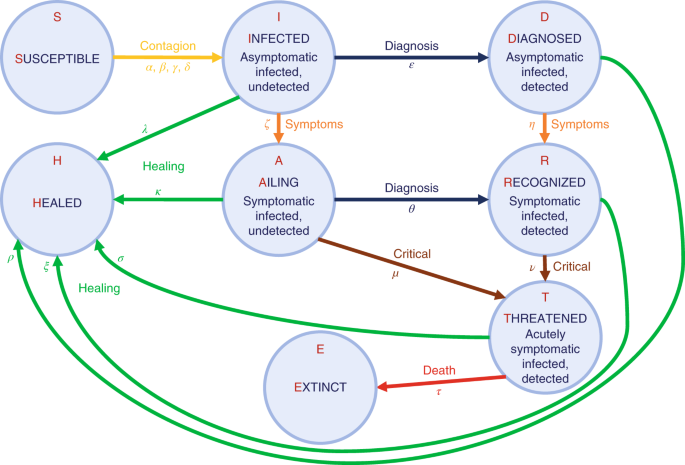
S_t the number of susceptibles in day t I_t the number of infectives in day t R_t. SIR model is of course a very simple model not suitable for shaping complex dynamics especially those which involve large and different populations. It examines how an infected population spreads a disease to a susceptible population which transforms into a recovered population. Of course for the SIR model we want the dependent variable names to be s i and r. Thus inoculation creates a direct path from the susceptible group to the recovered group without passing through the infected group see the diagram below. The SIR Model for Spread of Disease - Background. We consider two related sets of dependent variables. During the winter of 1968-1969 the United States was swept by a virulent new strain of influenza named Hong Kong flu for its place of discovery. The independent variable is time t measured in days.

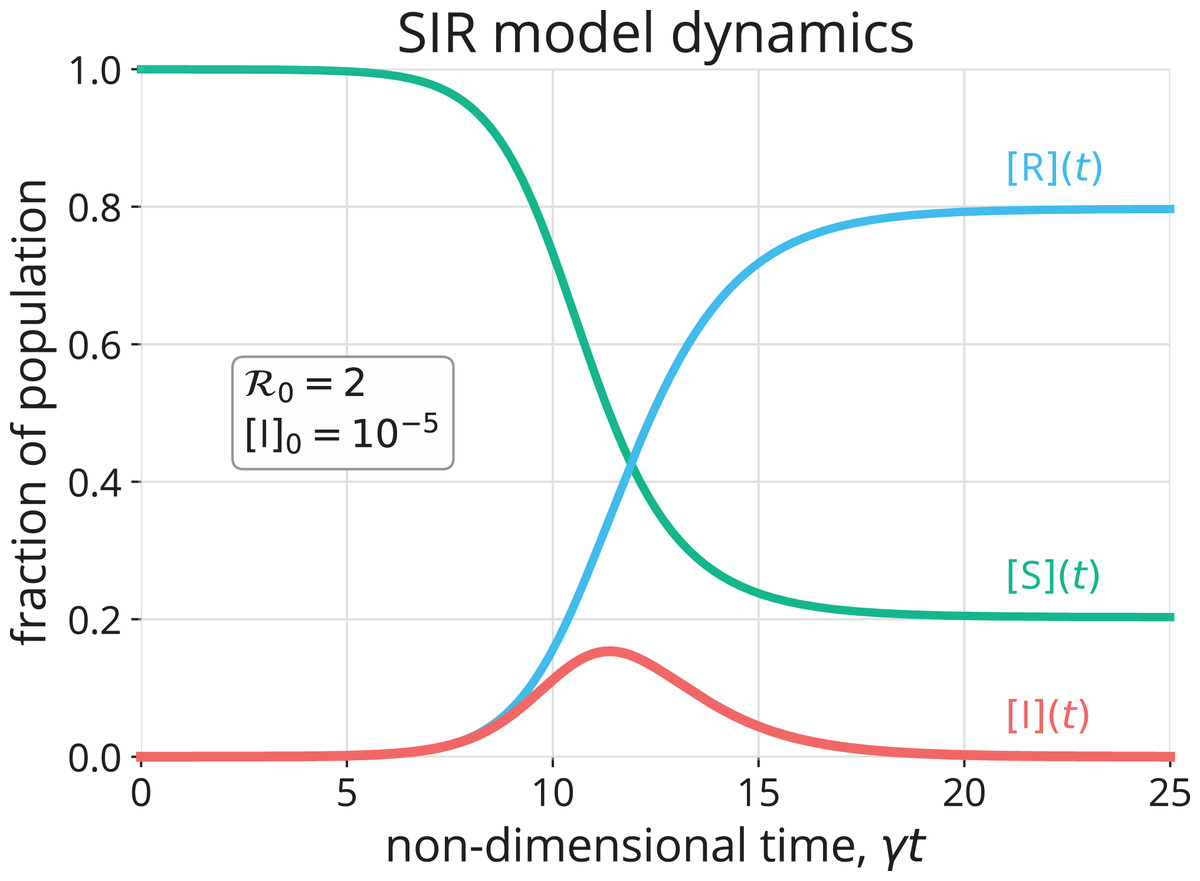
SIR model for COVID-19 According to this model and without any intervention to contain the spread the virus would be extinguished in about 180 days saving less than 20 of the population. The point of inoculation is to create herd immunity by stimulating in as many people as possible the antibodies that confer immunity -- but without actually giving those people the disease. The use of mathematics to model the spread of infectious disease is an increasingly critical tool not just for epidemiologists and health care providers. The SIR Model for Spread of Disease - Herd Immunity. The SIR model of an infectious disease The model I will introduce is the Susceptible Infected and Recovered SIR model. Compare your calculated value with the one you get by direct calculation from the definition c bk. Discrete SIR infectious disease model part 2.